会议时间:北京时间2021年12月18日-19日
会议地点:腾讯会议333-6927-1375
邀请专家:
|
桂贵龙 (湘潭大学、西北大学)
黄景炽(中山大学)
李岩岩(罗格斯大学)
|
郝成春(中科院数学所)
李维喜(武汉大学)
吕勇(南京大学)
|
|
牛冬娟(首都师范大学)
|
潜陈印(浙江师范大学)
|
|
孙永忠(南京大学)
王文栋(大连理工大学)
张挺(浙江大学)
|
王飞(上海交通大学)
张平(中科院数学所、国科大)
赵威任(纽约大学)
|
组委会:刘彦麟 熊金钢 许孝精 薛留堂
资助:“双一流”团队项目
会议日程
|
2021年12月18日 周六
|
|
时间
|
题目
|
报告人
|
主持人
|
|
8:50-9:00
|
开幕式:胡维副院长致辞
|
刘彦麟
|
|
9:00-9:50
|
Anisotropic Caffarelli-Kohn-Nirenberg type inequalities
|
李岩岩
罗格斯大学
|
|
9:50-10:40
|
Enhanced dissipation for the third component of 3D anisotropic Navier-Stokes equations
|
张平
中科院数学所、国科大
|
许孝精
|
|
10:50-11:40
|
Asymptotic Stability of Boussinesq Equations without Thermal Conduction
|
孙永忠
南京大学
|
|
午 休
|
|
14:00-14:50
|
Lagrangian approach to global well-posedness of the viscous surface wave equations
|
桂贵龙
湘潭大学、西北大学
|
许孝精
|
|
14:50-15:40
|
Global weak solutions to a 2D compressible non-resistivity MHD
|
张挺
浙江大学
|
|
15:50-16:40
|
Well-posedness of Linearized Incompressible Ideal MHD with Closed Free Surfaces
|
郝成春
中科院数学所
|
薛留堂
|
|
16:40-17:30
|
Long-time behavior of large solutions with just bounded density to compressible Navier-Stokes equations
|
黄景炽
中山大学
|
|
2021年12月19日 周日
|
|
时间
|
题目
|
报告人
|
主持人
|
|
9:00-9:50
|
Homogenization of Poisson and Stokes equations in the whole space
|
吕勇
南京大学
|
熊金钢
|
|
9:50-10:40
|
Caffarelli-Kohn-Nirenberg's regularity theory for the 3D Navier-Stokes equations: its generalization and application
|
王文栋
大连理工大学
|
|
10:50-11:40
|
Gevrey well-posedness of Prandtl and MHD boundary layer equations
|
李维喜
武汉大学
|
|
午 休
|
|
14:00-14:50
|
On the Euler+Prandtl expansion for the Navier-Stokes equations
|
王飞
上海交通大学
|
刘彦麟
|
|
14:50-15:40
|
Enhanced dissipation for the 2D Couette flow
|
赵威任
纽约大学
|
|
15:50-16:40
|
Global well-posedness for the 2-D Boussinesq system with variable viscosity
|
牛冬娟
首都师范大学
|
薛留堂
|
|
16:40-17:30
|
Global well-posedness for 3D incompressible inhomogeneous asymmetric fluids
|
潜陈印
浙江师范大学
|
报告题目和摘要
Lagrangian approach to global well-posedness of the viscous surface wave equations
桂贵龙
湘潭大学、西北大学
Abstract:In this talk, we investigate the global well-posedness of the classical viscous surface waves in the absence of surface tension effect with the reference domain being the horizontal infinite slab. The fluid dynamics are governed by the gravity-driven incompressible Navier-Stokes equations. Even though Lagrangian formulation is most natural to study free boundary value problems for incompressible flows, few mathematical works for global existence are based on such an approach in the absence of surface tension effect, due to breakdown of Beale's transformation. We develop a mathematical approach to establish global well-posedness based on the Lagrangian framework by analyzing suitable "good unknowns" associated with the problem, which requires no nonlinear compatibility conditions on the initial data.
Well-posedness of Linearized Incompressible Ideal MHD with Closed Free Surfaces
郝成春
中科院数学与系统科学研究院
Abstract:In this talk, I review some results of free boundary problem of incompressible ideal MHD in a bounded domain with closed free surfaces based on the joint works with Prof. T. Luo, especially the well-posedness for the linearized system. We expressed the magnetic field in terms of the velocity field and the deformation tensors in the Lagrangian coordinates, and substituted the magnetic field into the momentum equation to get an equation of the velocity in which the initial magnetic field serves only as a parameter. Then, we linearized this equation with respect to the position vector field whose time derivative was the velocity, and obtained the local-in-time well-posedness of the solution by using energy estimates of the tangential derivatives and the curl with the help of Lie derivatives and the smooth-out approximation.
Long-time behavior of large solutions with just bounded density to compressible Navier-Stokes equations
黄景炽
中山大学
Abstract:In this talk, we will focus on the long-time behavior of large solutions with just bounded density (without vaccum) for compressible Naiver-Stokes equations. This result removes the Hölder condition of previous result.
Gevrey well-posedness of Prandtl and MHD boundary layer equations
李维喜
武汉大学
Abstract:We establish the well-posedness of the Prandtl and MHD boundary layer system in Gevrey function spaces. By observing new type of cancellation mechanism in the system for overcoming the loss derivative degeneracy, we show that the Prandtl and MHD boundary layer system are well-posed in Gevrey space without any structural assumption.
Anisotropic Caffarelli-Kohn-Nirenberg type inequalities
李岩岩
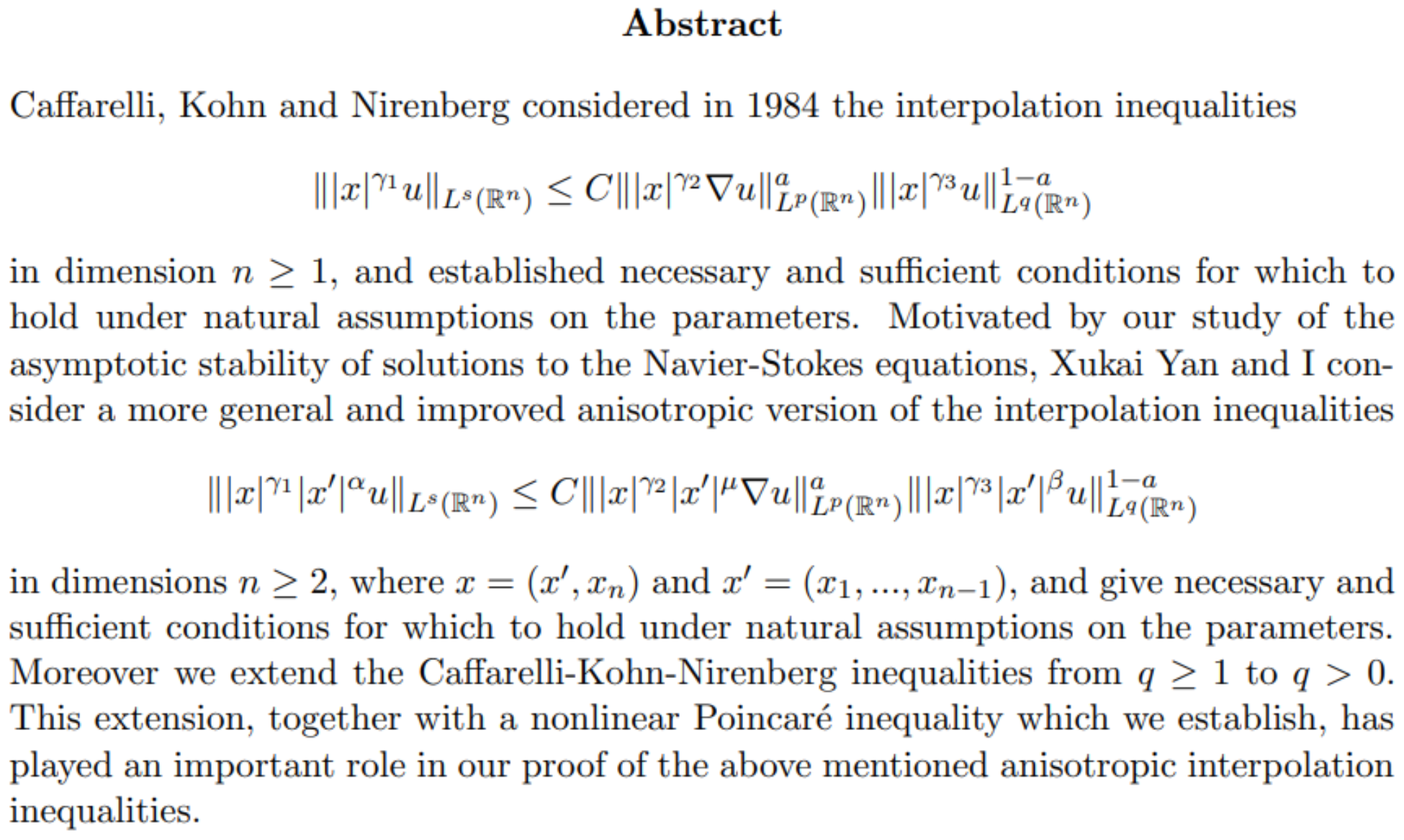
罗格斯大学
Homogenization of Poisson and Stokes equations in the whole space
吕勇
南京大学
Abstract:We consider the homogenization of the Poisson and the Stokes equations in the whole space perforated with periodically distributed small holes. The periodic homogenization in bounded domains is well understood, following the classical results of Tartar, Cioranescu-Murat, Allaire in 80s and 90s. In this paper, we show that these classical homogenization results in a bounded domain can be extended to the whole space R^d. Our results cover all three cases corresponding to different sizes of holes and cover all d\geq 2.
Global well-posedness for the 2-D Boussinesq system with variable viscosity
牛冬娟
首都师范大学
Abstract:In this talk, we investigate the global well-posedness of 2-D Boussinesq system, which has variable kinematic viscosity yet without thermal conductivity and buoyancy force, provided that the viscosity coefficientis sufficiently close to some positive constant in L∞. In addition, the decay estimate of the velocity fields is also obtained.It is a joint work with Lu Wang.
Global well-posedness for 3D incompressible inhomogeneous asymmetric fluids
潜陈印
浙江师范大学
Abstract:In this paper, we investigate the 3D inhomogeneous incompressible asymmetric fluids system with density-dependent viscosity. By the assumption of the smallness of initial velocity in the critical Besov space and the initial density in the critical Besov space and bounded away from vacuum, the local and global well-posedness of 3D inhomogeneous incompressible asymmetric fluids is obtained. By giving the different estimates for pressure, it shows the a priori estimate for the corresponding linearized equation. This not only improves the previous results for 3D inhomogeneous incompressible Navier-Stokes equations with density-dependent viscosity, but also obtains the new results on the micropolar system without smallness for density in critical Besov spaces.
Asymptotic Stability of Boussinesq Equations without Thermal Conduction
孙永忠
南京大学
Abstract:We consider the motion of viscous incompressible fluids under the action of gravitation/buoyancy modeled by the Boussinesq equations in the absence of thermal conduction which is a simple elliptic-parabolic-hyperbolic coupled nonlinear system. The fluid motion is assumed to be in an infinite stripe domain in two or three dimensional space. The asymptotic stability is proved for a specific stationary solution in contrast to Rayleigh-Taylor instability. Moreover, we also show exact decay rates of the solutions to the perturbed system with small initial dara. This is a joint work with Lihua Dong.
On the Euler+Prandtl expansion for the Navier-Stokes equations
王飞
上海交通大学
Abstract: We establish the validity of the Euler+Prandtl approximation for solutions of the Navier-Stokes equations in the half plane with Dirichlet boundary conditions, in the vanishing viscosity limit, for initial data which are analytic only near the boundary, and Sobolev smooth away from the boundary. Our proof does not require higher order correctors, and works directly by estimating an L 1 -type norm for the vorticity of the error term in the expansion Navier-Stokes−(Euler+Prandtl). An important ingredient in the proof is the propagation of local analyticity for the Euler equation, a result of independent interest.
Caffarelli-Kohn-Nirenberg's regularity theory for the 3D Navier-Stokes equations: its generalization and application
王文栋
大连理工大学
Abstract:In this talk we'll recall Caffarelli-Kohn-Nirenberg's interior regularity theory and some generalized forms for the 3D Navier-Stokes equations. Moreover, we will show their important role in studying other properties of Navier-Stokes equations and some important applications for other models. This is a series of joint work with Professors Zhifei Zhang, Liqun Zhang, Di Wu, Jitao Liu, Tao Tao, Shuai Li, and others.
Enhanced dissipation for the 2D Couette flow
赵威任
纽约大学
Abstract:In this talk, I will first review some results about the enhanced dissipation for the 2D Couette flow. Then I will introduce a recent result about the nonlinear enhanced dissipation threshold in the cirtical space, where we proved the optimality of the size for the perturbations in critical space. This is a joint work with Li and Masmoudi.
Enhanced dissipation for the third component of 3D anisotropic Navier-Stokes equations
张平
中科院数学所、国科大
Abstract:In this talk, we study the decay rates for the global small smooth solutions to 3D anisotropic incompressible Navier-Stokesequations. In particular, we prove that the horizontal components of the velocity field decay like the solutions of 2D classical Navier-Stokes equations.While the third component of the velocity field decays as the solutions of 3D Navier-Stokes equations. We remark thatsuch enhanced decayrate for the third component is caused by the interplay between the divergence free condition of the velocity field and the horizontalLaplacian in the anisotropic Navier-Stokes equations.
Global weak solutions to a 2D compressible non-resistivity MHD
张挺
浙江大学
Abstract:In this talk, we consider a two-dimensional non-resistivity MHD system describing the evolution of viscous compressible and electrically conducting fluids under the action of a vertical magnetic field, with non-monotone pressure law and density-depending viscosity λ =λ(ρ). Using an approximate scheme and the compactness method which Bresch and Jabin proposed in (Bresch and Jabin, 2018), we prove the global existence of weak solutions. (Based on the work with Yu Liu)